Perspective Explained For Flat Earthers

Perspective is just Line-Of-Sight -- it doesn't change the lines-of-sight.

Perspective cannot make the bottom-half of an object disappear leaving the top in perfect proportion.

It simply does not work like this:

Figure 1. Flerfspective magically makes the ground rise but not objects!

When asked why the object would remain lower, Jack answered:

That object is vertical not horizontal

— Jack "The Carpenter" (@Adidas68Jack) October 11, 2017

It is because of the angle that Lines-Of-Sight make at the observer that things appear smaller and "lower" in the sky with increasing distance.  But, at no point, does 'perspective' ever change the actual lines-of-sight from object to observer.

Figure 2. How it works in reality is JUST simple line-of-sight

Mathematically, we can understand perspective is a very simple mapping of a 3D point [x, y, z] into 2D [x/z, y/z] by dividing the x and y parameters by z by taking the SLOPE of the lines (and slope and angle are basically the same thing).

For full 3D rendering you would need additional matrices for rotation about each axis and translation but if we already have the scene aligned where we want it then this is the essence of the process. We can see what happens just mapping a few points into 3D.

Let's orient our 3D space like so:

x-axis = left(-)/right(+)
y-axis = up(-)/down(+)
z-axis = behind(-)/forward(+)
viewer is [x=0, y=0, z=0]

Astute readers should recognize this as a slope (rise/run) and you can picture it as projecting the point onto the plane at z=1 by finding where a line from the observer to each point intersects the z=1 plane.

Figure 3. lines of sight in 2d basically just make a scaled image

Or in 3 dimensions with x/z and y/z slopes:

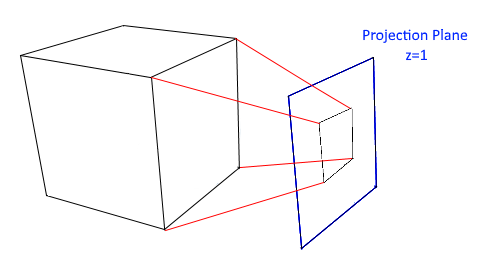Figure 4. Lines of sight in 3D work the same way

From this it is easy to see a more distant line would look progressively smaller in our field of view with an increase distance (z).

Consider a line from [0, 0, 1] (which just maps to 0,0 on our 2D grid because it's directly along our line of vision) to [0, -1, 1]  It is trivial to see that this line would be 1 unit high.  And then if we simply move it out to z=2 it becomes 1/2 unit high, and at z=4 it becomes 1/4 unit high.

[0, -1, 1] -> [0, -1]

[0, -1, 2] -> [0, -0.5]

[0, -1, 4] -> [0, -0.25]

Indeed,  we can see from this that each time we double our distance we cut the apparent size in half.

This is the 'Law of Perspective' that Flat Earthers go on about without understanding it.

We can also see from this that if a flat and level surface like the water were actually flat it could NEVER hide the bottoms of buildings and mountains as we observe with increased distance which we see over...  Because x=0, y=0 is along our line of vision and a positive y divided by a positive z will NEVER become a negative y value, so it would not be possible for it to block our line of sight towards any distant point above that line of vision.

And we see the impact of curvature over...

and over...

and over...

and over...

and over...

and over...

and over...

Zoom

Flat Earthers claim zoom will magically bring this back we can see here that zoom does nothing to bring back the bottoms of the buildings which are over the horizon.  And I've shown above that this cannot be explained away as perspective because that isn't how perspective works.

But does Zoom actually work?

No, it very clearly doesn't.

What Flat Earthers have done is confused a ship being too small for their eye or some camera to RESOLVE with the ship actually going over the horizon.  For example, this video shows a boat that is very near the horizon line but NOT appreciably past the horizon line.

And they claim this is "100% proof" - it's just absurd levels of Dunning-Kruger on display in the Flat Earth community.  They also usually ignore the fact that since their camera lens isn't half-way under the water the 'drop' calculation alone tells you nothing.  You have to know the exact distance, and you have to take into account the elevation of the observer, and you have to account for the refraction of the air at that time to get a good estimate for distance to the horizon.

Unless you get down pretty low to the ground and have an extremely clear day it's very hard to verify that the ship is, in fact, going over the horizon.  You can see this series here the water level is rising as we scale and align the same point on the ship.  Now this COULD be refraction - I can't 'prove' it with this image series because the effect size here is too small.  But you cannot miss it when 2/3 of the mountain is missing as I showed in the series above.

Refraction

From Andrew T Young's papers on Ray Bending and Refraction we know that the ray curvature on the Earth is about 15% of Earth's curvature in a normal atmosphere.

In free convection, the (adiabatic) lapse rate is about 10.6°/km or γ = 0.0106 K/m. The numerator of the formula above becomes .034 − .0106 = .0234, so the ratio k is about 1/6.6 or 0.152. In other words, the ray curvature is about 15% that of the Earth; the radius of curvature of the ray is about 6.6 times the Earth's radius. This is close to the condition of the atmosphere near the ground in the middle of the day, when most surveying is done; the value calculated is close to the values found in practical survey work.

This is also right around the value used for surveying adjustments when proper optical methods cannot be used (surveying both directions, under the same conditions, will help negate out both refraction and Earth curvature, this is called Reciprocal Leveling).

Image Credit: http://www.aboutcivil.org/curvature-and-refraction.html

However, despite the name 'Standard Refraction', we rarely encounter exactly such ideal conditions, as any text on Geodesy will affirm.  Without exact data about the atmosphere at the time of observation, the best we can do is approximate it.

That's why my horizon calculator allows you to enter refraction.

Conclusion

In the worst cases I find that about 15% refraction is sufficient to account for observations - and only rarely is that much needed,  Meanwhile, Flat Earthers fail to account for 100% of the missing mountains - they falsely appeal to 'Perspective' as the answer but they are unable to explain how it manages to do this (and I have shown here that it cannot).

I appeal to refraction for the small amount of difference between a pure sphere and the reality of what we see on Earth.  If we didn't know it was refraction we would think the Earth was about 15% larger than it is -- but we KNOW that is wrong because we know how to measure it more carefully than these gross observations made by Flat Earthers.